6174 constant | Kaprekar's Constant for 4

It essentially comes down to the clean bulk and the dirty bulk, which, as you’ve probably guessed, relates to the “cleanness” of the food you put into your body. Clean Bulking vs. Dirty Bulking. The key .

6174 constant*******The number 6174 is known as Kaprekar's constant after the Indian mathematician D. R. Kaprekar. This number is renowned for the following rule: Take any four-digit number, using at least two different digits (leading zeros are allowed).Arrange the digits in descending and then in ascending order to . Tingnan ang higit paThere can be analogous fixed points for digit lengths other than four; for instance, if we use 3-digit numbers, then most sequences (i.e., other than repdigits such as 111) will . Tingnan ang higit pa• Bowley, Roger. "6174 is Kaprekar's Constant". Numberphile. University of Nottingham: Brady Haran.• Sample (Perl) code to walk any four-digit number to Kaprekar's Constant• Tingnan ang higit pa

• 6174 is a 7-smooth number, i.e. none of its prime factors are greater than 7.• 6174 can be written as the sum of the first three powers of 18:• The . Tingnan ang higit pa

Kaprekar constant, or 6174, is a constant that arises when we take a 4-digit integer, form the largest and smallest numbers from its digits, and then subtract these two numbers. Continuing . Applying the Kaprekar routine to 4-digit number reaches 0 for exactly 77 4-digit numbers, while the remainder give 6174 in at most 8 iterations. The value 6174 is sometimes .

Kaprekar's Constant is 6174. Take a 4-digit number (using at least two different digits) and then do these two steps around and around: • Arrange the digits in descending order • Subtract the . 6174, known as Kaprekar's constant, is a fixed point of this algorithm. Any four-digit .6174 constant Kaprekar's Constant for 4 The value 6174 is sometimes known as Kaprekar's constant (Deutsch and Goldman 2004). This pattern breaks down for 5-digit numbers, which may converge to 0 or .

Kaprekar's constant, or 6174, is a constant that arises when we take a 4-digit integer, form the largest and smallest numbers from its digits, and then subtract these two . The number 6174 is a really mysterious number. At first glance, it might not seem so obvious. But as we are about to see, anyone who can subtract can uncover the mystery that makes 6174 so special. Kaprekar's operation. In .Kaprekar's constant of 6174 is notable for the following property: 1) Take any four-digit number with at least two digits different. 2) Arrange the digits in ascending and then in descending . It’s a simple mathematical algorithm discovered by Indian mathematician D. R. Kaprekar. It shows that subtracting two numbers in a repeating cycle yields the same result of . 6174 is known as Kaprekar’s constant as it was invented by Indian mathematician DR Kaprekar in 1949.. www.youtube.com. Even mathematicians, till date, aren’t sure how to explain this magic number.Kaprekar's Constant is 6174. Take a 4-digit number (using at least two different digits) and then do these two steps around and around: • Arrange the digits in descending order • Subtract the number made from the digits in ascending order. and we will eventually end up with 6174. Example: 1525 5521 - 1255 = 4266 6642 - 2466 = 4176 7641 .Gráfico que muestra la rutina con la que se obtiene la constante de Kaprekar 6174. El algoritmo es el siguiente: [3] Elíjase cualquier número natural en una base; Créese un nuevo número ordenando las cifras de en orden descendente; y un nuevo número , con el orden de sus cifras ascendente.; Repítase el paso anterior con el resultado de ; La secuencia creada por los .

6174 constant 6174 is also known as Kaprekar's Constant.More links & stuff in full description below ↓↓↓This video features University of Nottingham physics professor Roge.

Kaprekar's constant is 6174: Proof without calculation. 7. What is the logic behind Kaprekar's Constant? Related. 9. Every integer is congruent to the sum of its digits mod 9. 4. Ternary Expansion of $1/4$ 1. Multiples of 11 in a Fibonacci-like sequence formed by concatenation instead of addition. 0. The value 6174 is sometimes known as Kaprekar's constant (Deutsch and Goldman 2004). This pattern breaks down for 5-digit numbers, which may converge to 0 or one of the 10 constants 53955, 59994, 61974, 62964, 63954, 71973, 74943, 75933, 82962, 83952. In 1949, a mathematician named D.R. Kaprekar discovered an intriguing number sequence that would later be known as Kaprekar’s Constant.The constant, which is 6174, is a four-digit number that .

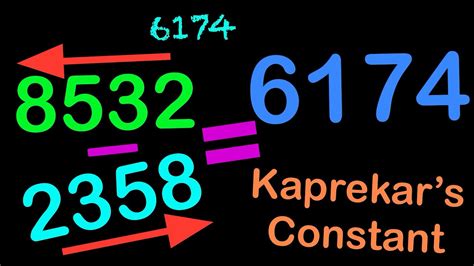
8532 – 2358 = 6174. Again, if we choose the current year, 2018, we get the sequence. 8210 – 0128 = 8082. 8820 – 0288 = 8532. 8532 – 2358 = 6174. The iterative process always converges to Kaprekar’s constant in, at most, seven steps. Once this fixed point is reached, the process will simply cycle, yielding 7641 – 1467 = 6174.

le nombre constant zéro, . Diagramme de transition par la transformation de Kaprekar de nombres à quatre chiffres aboutissant au nombre fixe 6174. Là encore, avec une définition stricte de la transformation, le diagramme comprend moins d'états : les bulles contenant des nombres incluant un chiffre nul sont retirées, et les nombres à .Kaprekar's Constant for 4Il numero 6174 è conosciuto come la costante di Kaprekar [1] [2] [3] in onore del matematico indiano Dattatreya Ramachandra Kaprekar che la scoprì. Tale numero possiede la seguente proprietà: . (EN) Eric W. Weisstein, Kaprekar's Constant, su MathWorld, Wolfram Research. Mysterious Number 6174 Article, su plus.maths.org. Now how about making Kaprekar music. There are some wonderful attempts to convert the digits of mathematical constant Pi to musical sequence [check Michael John Blake- A musical interpretation of pi, and Lucy .

6174 - Kaprekar's Constant: Test in Python. Oct 31, 2021 3 min read 6174 - doesn't seem like a very interesting number at first glance but this Numberphile video shows that all four digit numbers converge to this number if the following procedure is followed: Start with a four digit number. . 6174 parece un número cualquiera, salido del aire, sin ninguna credencial para la fama. Sin embargo, lleva intrigando a matemáticos y entusiastas de la teoría de los números desde 1949.Kaprekar's Constant for 4-Digit Numbers: 6174. Kaprekar's constant of 6174 is notable for the following property:. 1) Take any four-digit number with at least two digits different. 2) Arrange the digits in ascending and then in descending order to get two four-digit numbers, adding leading zeros if necessary.Because they're kind of arbitrary. We get 6174 in base 10. We get the cycles (2256, 5312, 3856) and (3712, 5168, 5456) in base 9. It's just some numbers. It's not that 6174 is special any more than like "36 is an amazing number cause it's the area of the square with side length 6". Sure, but side length 6 is a hell of a restriction.

It’s a simple mathematical algorithm discovered by Indian mathematician D. R. Kaprekar. It shows that subtracting two numbers in a repeating cycle yields the same result of 6174 every time. If. The Kaprekar constant 6174. August 29, 2011 GB High School Mathematics, High School Number Theory. In the mysterious 495, (1) we chose any 3-digit number, (2) arranged the digits in decreasing order forming the largest integer, (3) arranged the digit in increasing order forming the smallest integer, and (4) subtracted the smaller from the .Working largely alone, Kaprekar discovered a number of results in number theory and described various properties of numbers. [3] In addition to the Kaprekar's constant and the Kaprekar numbers which were named after him, he also described self numbers or Devlali numbers, the harshad numbers and Demlo numbers.He also constructed certain types of magic squares .

Savannah Bond, Kay Lovely - Look Out Below 09/23/22 Screenshots: FileInfo: 225 MiB - 23mn 10s - mp4 - 854x480 . Gemini37 PlanetSuzy's Halloween/Horror Music Thread PASS for RAR: drdoom-psuzy If any of my links is dead, please PM me and I .

6174 constant|Kaprekar's Constant for 4